Sin60

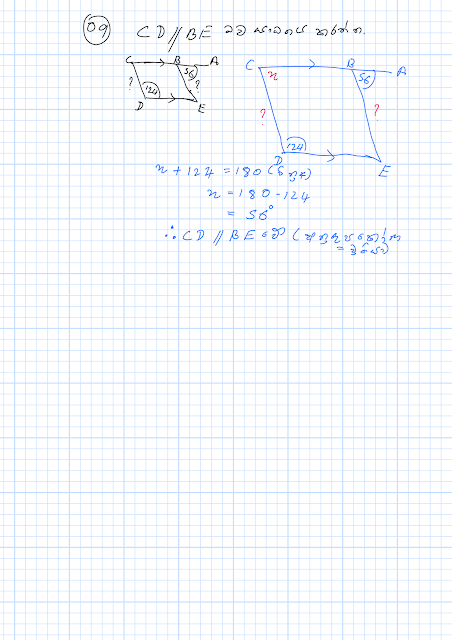
ත්‍රිකෝණ අංගසාම්‍යය (Congruence of Triangles)

ත්‍රිකෝණ අංගසාම්‍යය යනු ජ්‍යාමිතියේ මූලික සංකල්පයක් වන අතර, ත්‍රිකෝණ දෙකක් හැඩයෙන් සහ ප්‍රමාණයෙන් හරියටම සමාන වන විට ඒවා අංගසාම්‍යය වේ යන්නයි. සරලව කිවහොත්, එක් ත්‍රිකෝණයක් අනෙක මතින් නියතව පිහිටුවිය හැකි නම්, ඒවා අංගසාම්‍යය වේ. අංගසාම්‍ය ත්‍රිකෝණවල අනුරූප පාදවල දිග සමාන වන අතර, අනුරූප කෝණවල විශාලත්වයද සමාන වේ. ත්‍රිකෝණ දෙකක් අංගසාම්‍යය දැයි තීරණය කිරීම සඳහා විවිධ ප්‍රමේයයන් (සාධක) ඇත:

1. පා.කෝ.පා. (SAS - Side-Angle-Side): ත්‍රිකෝණයක පාද දෙකක් සහ ඒවා අතර අන්තර්ගත කෝණය තවත් ත්‍රිකෝණයක අනුරූප පාද දෙකකට සහ අන්තර්ගත කෝණයට සමාන නම්.
2. පා.පා.පා. (SSS - Side-Side-Side): ත්‍රිකෝණයක පාද තුනේම දිග තවත් ත්‍රිකෝණයක අනුරූප පාද තුනේම දිගට සමාන නම්.
3. කෝ.කෝ.පා. (AAS - Angle-Angle-Side): ත්‍රිකෝණයක කෝණ දෙකක් සහ අන්තර්ගත නොවන පාදයක් තවත් ත්‍රිකෝණයක අනුරූප කෝණ දෙකට සහ අන්තර්ගත නොවන පාදයට සමාන නම්.
4. කර්.පා. (RHS - Right-angle, Hypotenuse, Side): සෘජුකෝණී ත්‍රිකෝණ දෙකක කර්ණය සහ එක් පාදයක් සමාන නම්.

අංගසාම්‍යය සංකල්පය ජ්‍යාමිතික ගැටලු විසඳීමේදී, විශේෂයෙන්ම නොදන්නා පාදවල දිග හෝ කෝණ සෙවීමේදී මෙන්ම ජ්‍යාමිතික සාධන (proofs) වලදී ඉතා වැදගත් මෙවලමකි.

Negative ions, also known as anions, are atoms or molecules that have gained one or more electrons, resulting in a net negative electrical charge. This occurs because electrons carry a negative charge, and by acquiring additional electrons, the total number of negative charges (electrons) exceeds the total number of positive charges (protons in the nucleus).

Here are the key characteristics and formation of negative ions:

• Formation: Anions are formed when a neutral atom or molecule accepts one or more electrons. This process is generally energetically favorable for non-metals, which have a high electron affinity (tendency to attract electrons).
• Charge: The charge of an anion indicates how many electrons it has gained. For example, a chloride ion (Cl−) has gained one electron, while an oxide ion (O2−) has gained two electrons.
• Electron Configuration: Anions often achieve a stable electron configuration, typically resembling that of a noble gas, by gaining electrons.
• Size: Anions are generally larger than their corresponding neutral atoms because the added electrons increase electron-electron repulsion and spread the electron cloud further from the nucleus.
• Nomenclature:
  • For monatomic anions (single atom), the ending of the element's name is changed to "-ide" (e.g., Chlorine becomes Chloride, Oxygen becomes Oxide).
  • Polyatomic anions (ions made of multiple atoms) often have names ending in "-ate" or "-ite" (e.g., Sulfate, Sulfite, Nitrate, Nitrite), indicating the presence of oxygen, or "-ide" for some simple ones (e.g., Hydroxide).

Negative ions are crucial in countless chemical reactions, playing a vital role in forming ionic compounds, conducting electricity in solutions, and facilitating biological processes. They are attracted to positive ions, forming the basis of ionic bonding and the structure of many salts.

What specific charge of negative ions would you like a list for? For example, -1 ions, -2 ions, etc.

Negative ions, also known as anions, are atoms or molecules that have gained one or more electrons, resulting in a net negative electrical charge. With extra negatively charged electrons, they have more electrons than protons. Common examples include:

These charged particles are fundamental to many chemical and biological processes, including the formation of ionic compounds, the conduction of electricity in solutions, and nerve impulses in living organisms. The attraction between oppositely charged ions is what drives the formation of ionic bonds.

+1ions 

Here is a list of common +1 ions (cations with a single positive charge):

(Note: This is a diatomic ion, consisting of two mercury atoms with an overall +2 charge, so each mercury atom effectively has a +1 charge.)

                                                     +2 ions

Here is a list of common +2 ions (cations with a double positive charge), also known as divalent cations:

Here is a list of common +3 ions (cations with a triple positive charge), also known as trivalent cations: